The Senior Maths Challenge is a 90-minute paper given to students usually around year 10-13 by the United Kingdom Maths Trust. It consists of 25 multiple-choice questions of increasing difficulty, and the top scoring students are sent to do the British Mathematical Olympiad Round 1, and then 100 of the top scoring students from that are sent to do the British Mathematical Olympiad Round 2, and then the top students, if eligible to represent the UK, are sent to the UK’s IMO training camp. In year 9, I narrowly slipped into the BMO1 threshold by a 1-point margin, and was 3 points away from the BMO2, which to solve one question from is my goal this year. But before I can do the BMO, I must do the SMC, which happened, at the time of writing, yesterday. Let’s do a super fast speedrun through the questions and do some math for fun!
Video of me explaining solutions coming soon.
Contents
Page 1 (Questions 1-9)
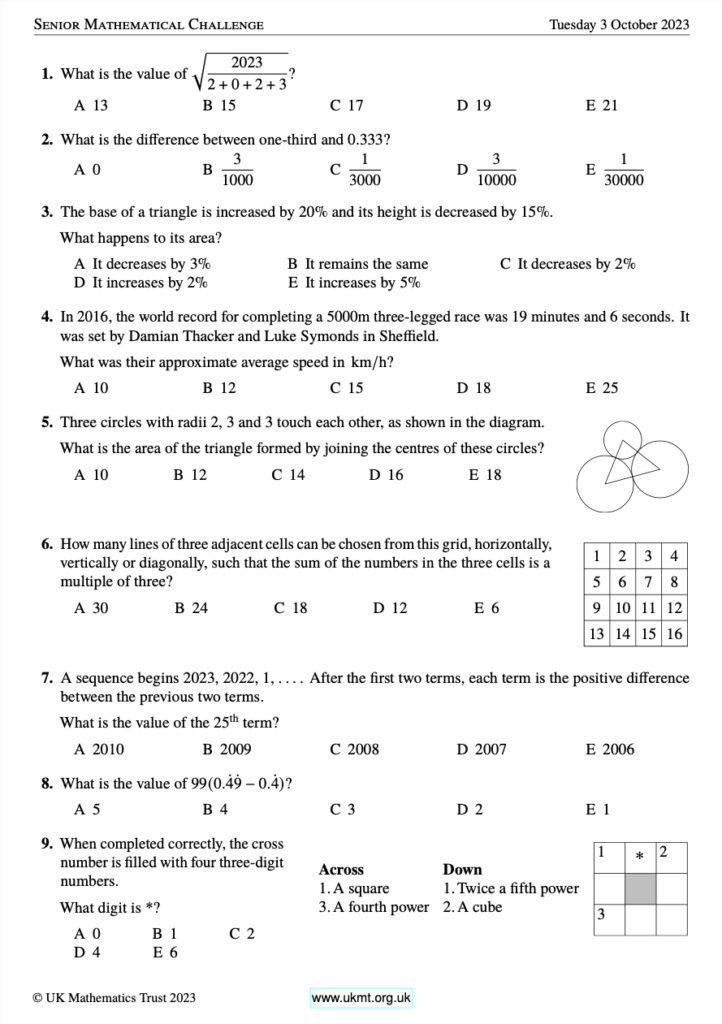
Question 1: Basic math. \(2023 = 7\cdot 17^2\), so the answer is C.
Question 2: Basic math once again. \(\frac{1}{3} – 0.333 = 0.000\overline{3} = \frac{1}{3} \times \frac{1}{1000} = \frac{1}{3000}\) so the answer is C.
Question 3: The area of a triangle is proportional to its base times its height. An increase of 20% is 1.2, and a decrease of 15% is 0.85, so \(1.2 \times 0.85 = 1.02\) with some converting to fractions, so the answer is D.
Question 4: 19 minutes and 6 seconds is close enough to 20 minutes, which is a third of an hour, so \(5 \div \frac{1}{3} = 15\), so the answer is C.
Question 5: The resulting triangle has side lengths 5, 5, and 6. I used Heron’s formula sitting the paper, but you can also draw a tangent that goes through the intersection point of the two larger circles and split the triangle into 2 3-4-5 triangles. Either way you get the answer of 12, B.
Question 6: Notice that from any two squares, if we go to either of the 8 adjacent squares, the difference between the numbers is constant. For example, if we go down a square from 6 and 4, the ending square increases by 4 to 10 and 8. If we look at any line of 3 cells, they sum to a multiple of 3, so moving the line in any direction will also result to a sum divisible by 3. Therefore all the lines work, and counting them there are 24.
Question 7: Writing down a few terms of the sequence, we get 2023, 2022, 1, 2021, 2020, 1, 2019, 2018…. Every third term goes down by 2, so the 25th term will be 2023 – 2×7 = 2007, which is D.
Question 8: \(0.\overline{49} – 0.\overline{4} = 0.050505… = 0.\overline{05} = \frac{5}{99},\) so the answer is 5, A.
Question 9: Down 1 has the least possibilities so let’s do that first. \(2^5 = 32, 3^5 = 243, 4^5 = 1024,\) so down 1 must be 243×2 = 486. Now across 3 must be \(5^4 = 625.\) Down 2 must then be $5^3 = 125$. There is only 1 square in the form 4*1, which is 441, so the answer is D.
Page 2 (Questions 10-13)
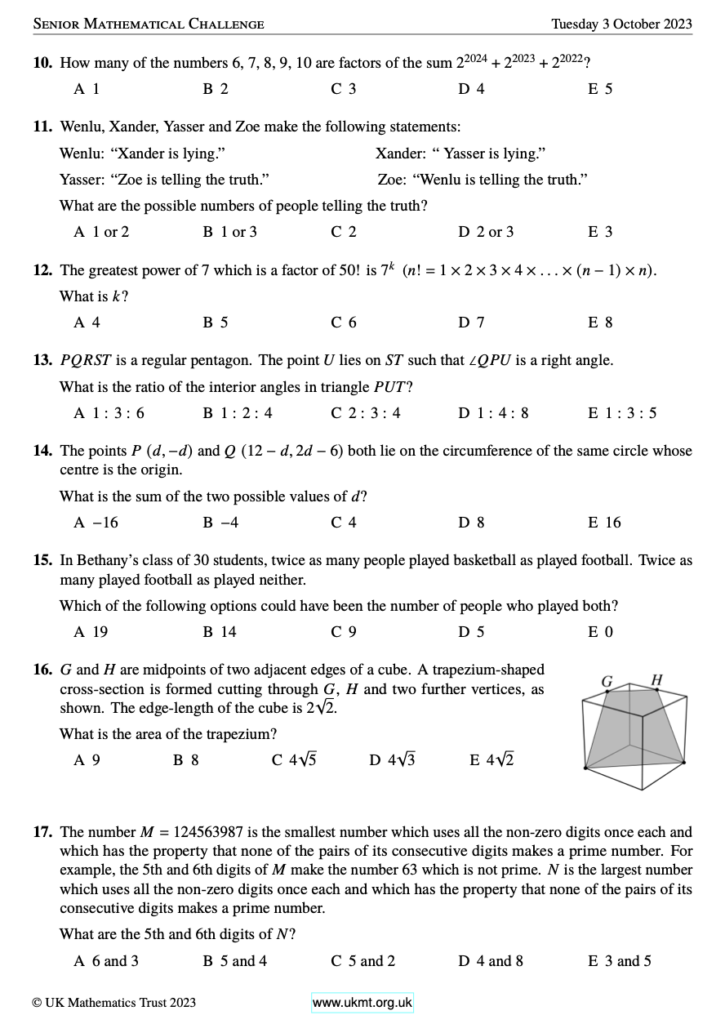
Question 10: Factorising the expression gives us \(2^{2022}(2^0 + 2^1 + 2^2) = 2^{2022}\cdot 7\). This means that 7 and 8 are factors, so the answer is B.
Question 11: We can make a table for who is telling the truth if we change who we assume is telling the truth. It would look like this:
| Wenlu | Xander | Yasser | Zoe | |
| Wenlu truth | T | L | T | T |
| Xander truth | L | T | L | L |
| Yasser truth | T | L | T | T |
| Zoe truth | T | L | T | T |
We see that there are either 1 or 3 people telling the truth, so the answer is B.
Question 12: 50 has 7 multiples of 7 in it, but 49 is 7 squared, so there are actually 8 7’s in 50!, so the answer is E.

Question 13: The interior angle of a pentagon is \(180 – \frac{360}{5} = 108^\circ\). Therefore angle \(\angle UPT = 18^\circ\). Angle \(\angle TUP = 108^\circ,\) so angle \(\angle UPT = 180 – 18 – 108 = 54^\circ\). Therefore the ratio is \(1 : 3 : 6\), so the answer is A.
Question 14: Using the Pythagorean Theorem,
\(d^2 + d^2 = (12 – d)^2 + (2d – 6)^2\)
\(2d^2 = 144 – 24d + d^2 + 4d^2 – 24d + 36\)
\(2d^2 = 5d^2 – 48d + 180\)
\(3d^2 – 48d + 180 = 0\)
\(d^2 – 16d + 60 = 0\)
By Vieta’s formulas, the sum of the roots is 16, so the answer is E.
Question 15: I trial and errored this question. Solution coming soon.
Question 16: Using Pythagoras, GH = 2 and the base length is 4. The height can be found using 3D Pythagoras, note that it is NOT \(2\sqrt{2}\) as it is slanted:
\(h = \sqrt{(2\sqrt{2})^2 + 2\cdot \frac{\sqrt{2}}{2}^2}\)
\(= \sqrt{8 + 2\cdot \frac{1}{2}}\)
\(= \sqrt{9} = 3.\)
So the area is 3×3 = 9, so the answer is A.
Question 17: Trial and error once again. I knew that the first 4 digits would be 9876, so tried the options from largest to smallest.